Appendix C
Directional Wave Spreading
Functions
The directional spreading function, D(θ), describes the directional distribution
of wave energy in irregular multidirectional sea states. It can be quantified in
terms of the principal direction of wave propagation θp, and the directional spread
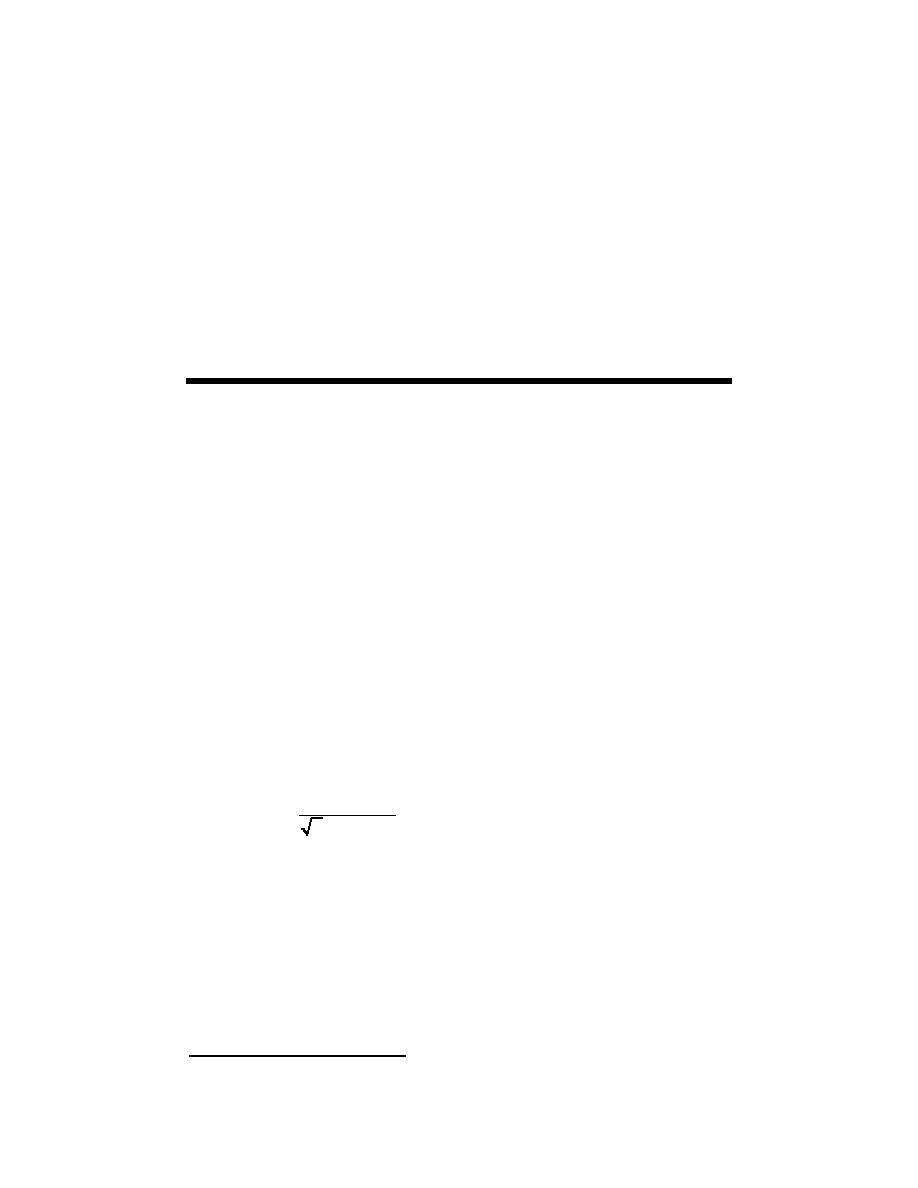
or standard deviation of the spreading function, σθ, which is defined as:
θp +π/ 2
∫
σθ
=
D(θ) (θ - θ p )2 dθ
2
(C1)
θp -π/ 2
A number of parametric shapes have been proposed to describe the directional
spreading function including the cosine-power, the circular normal, and wrapped-
normal distributions. These are described in the following paragraphs.
Cosine-Power Spreading Function
The cosine-power function is an extended version of the cosine-squared direc-
written as:
Γ(s + 1)
D(θ) =
cos2s (θ - θ p )
for | θ - θ p | < π / 2
(C2)
π Γ(s + 1/ 2)
where Γ is the gamma function. The parameter s is an index describing the degree
of directional spreading with s → ∞ representing a unidirectional wave field.
Circular-Normal Spreading Function
The circular normal distribution was proposed by Borgman (1969) and can be
written as:
1
References cited in this appendix are listed in the References at the end of the main text.
C1
Appendix C Directional Wave Spreading Functions



 Previous Page
Previous Page
