Fundamentals of Engineering Design
The concentration calculated from Brownlie's sediment transport equation applies only vertically
above the bed. The total sediment transport, computed in SAM, in weight per unit time is computed by
the following equation:
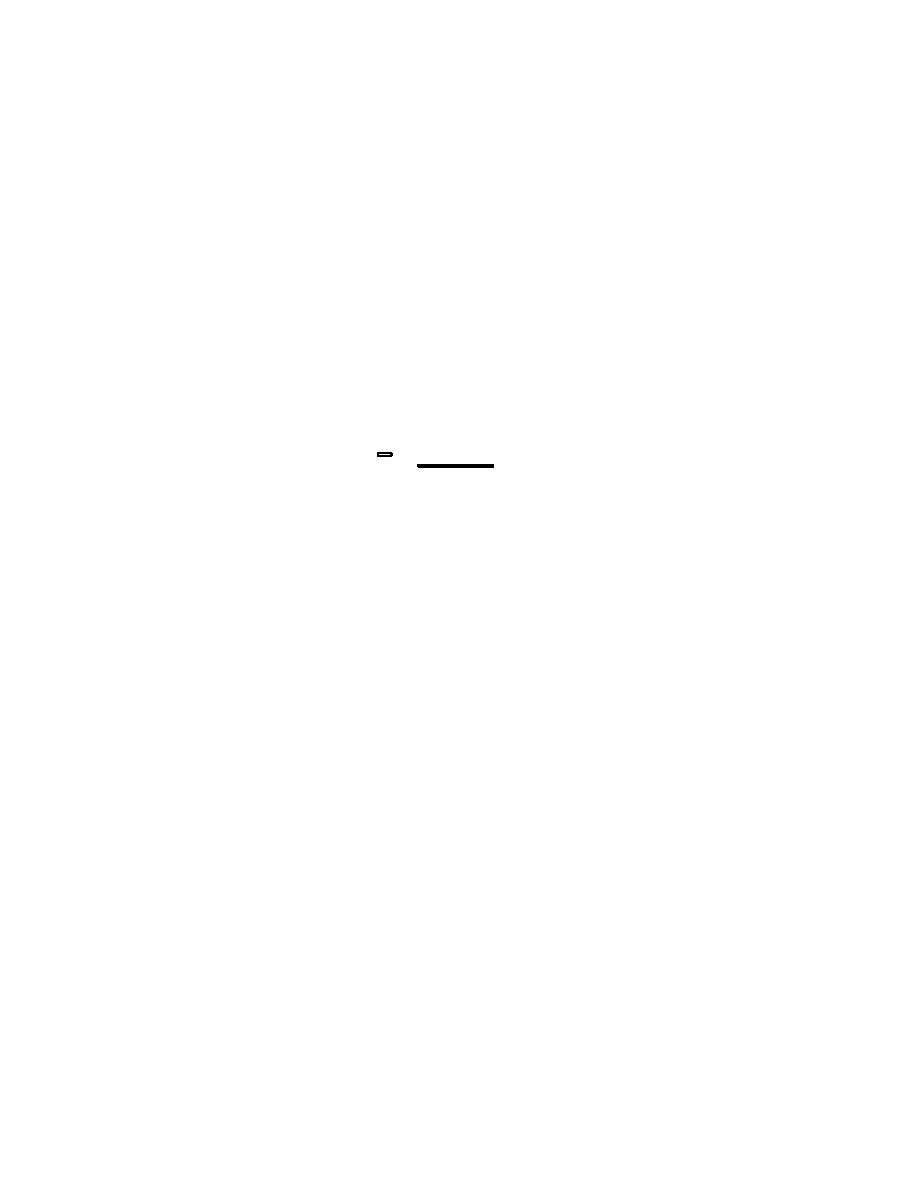
Qs ' ( C B D V
(5.19)
where: Qs
=
sediment transport in weight/time;
B
=
base width;
=
specific weight of water;
C
=
concentration;
D
=
hydraulic depth; and
V
=
average velocity.
An average concentration for the total discharge is then calculated by:
Qs
C'
(5.20)
0.0027 Q
where: C = concentration using the total discharge in ppm;
Qs = sediment transport in tons/day; and
Q = discharge in ft3/s.
5.3.6.5 Model Application
Copeland's stable channel design method simultaneously solves Eqs. (5.11), (5.12), and (5.14)
to satisfy water and sediment continuity for a series of slopes and widths. The designer may then select any
point along a curve plotted with width on the x-axis and slope on the y-axis. The minimum slope can be
selected as an extremal hypothesis design according to Chang (1980):
For an alluvial channel, the necessary and sufficient condition of equilibrium occurs
when the stream power per unit length of channel QS is a minimum subject to given
constraints, where = the specific weight of water; Q = discharge; and S = slope.
Hence, an alluvial channel with water discharge Q and sediment load Q as
s
independent variables tends to establish its width, depth and slope such that QS is
a minimum. Since Q is a given parameter, minimum QS also means minimum
channel slope.
If the minimum slope design is desired it can be evaluated graphically using a stable channel curve. A stable
channel curve is a plot of slope versus width, in which the minimum stream power design corresponds to
minimum slope. An example of a stable channel curve is given in Figure 5.43.
179



 Previous Page
Previous Page
