Fundamentals of Engineering Design
Fg is the grain Froude number representing the square root of the ratio of drag forces on a particle
to the particle weight. Brownlie defines the grain Froude number by the following equation (Brownlie,
1981):
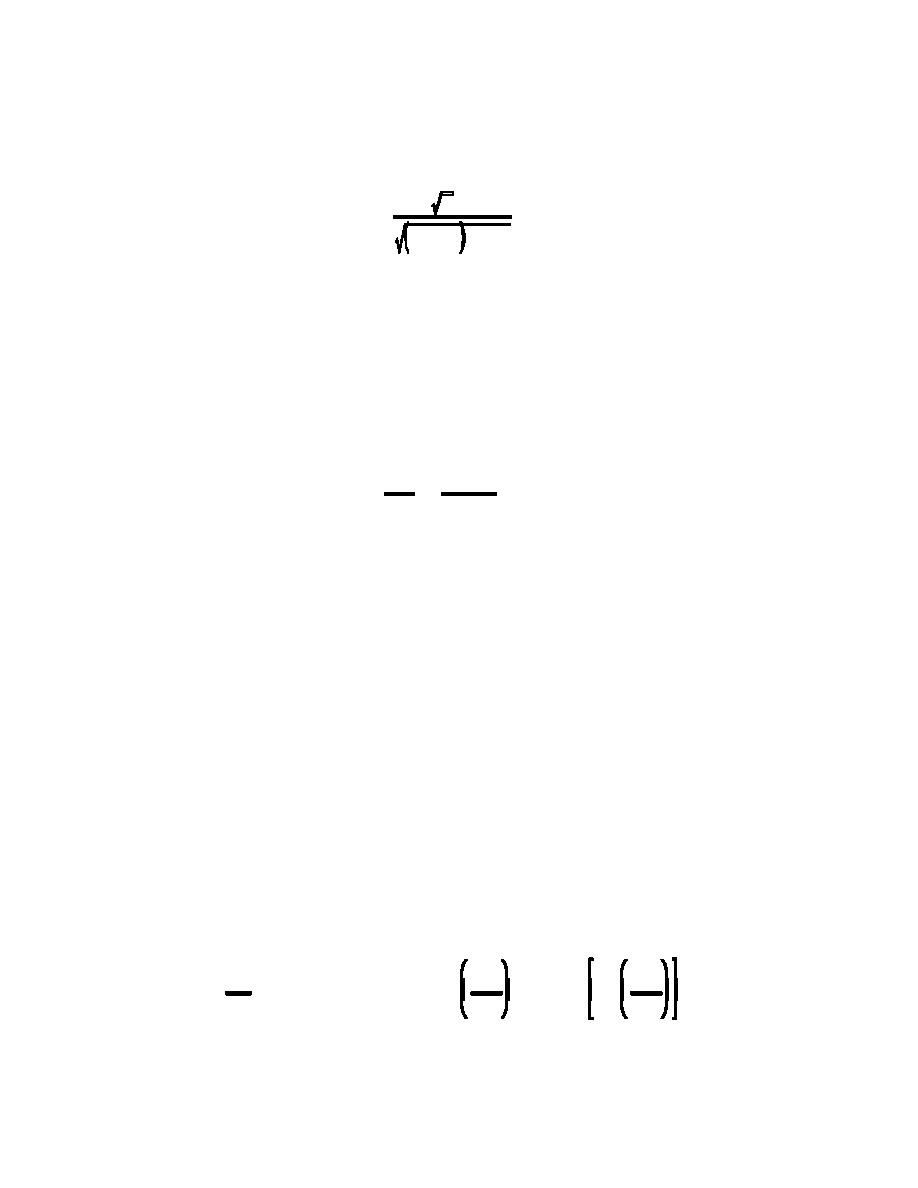
DV
Fg '
(5.6)
Ds & D gD50
where:
=
density of water;
s
=
density of sediment particles;
V
=
depth average velocity;
D50
=
median grain size of the particles; and
g
=
acceleration due to gravity.
D50/ is the ratio of the mean grain size of the particles to the thickness of the laminar sublayer. It
is defined by:
D50
u( D50
(5.7)
'
11.6 <
*
where:
= laminar sublayer thickness;
u*
= shear velocity; and
= kinematic viscosity of water.
Brownlie plotted the grain Froude number versus the slope for all upper and lower regime data to
incorporate the slope, Figure 5.41. Brownlie reported that beyond a slope of S = 0.006, only the upper
flow regime exists. For values lower than 0.006 an approximate dividing line in the data may be defined
by Fg = Fg . Fg is computed from the following regression analysis as developed from Figure 5.41.
)
)
F gN ' 1.74 Sf&0. 33
(5.8)
Brownlie plotted the Fg /Fg versus D50/ for transitional data with slopes less than 0.006. Division of Fg
)
by Fg eliminates the bias found when plotting Fg against just the slope. This defines the transition between
)
upper and lower regime as seen in Figure 5.42. The transition region is defined by Eqs. (5.7) and (5.8)
for the lower and upper limits of the flow regimes.
For the lower limit of the upper flow regime, D50/ < 2:
2
Fg
D50
D50
log
' &0.02469 % 0.1517 log
% 0.8381 log
(5.9)
*
*
Fg)
and log 1.25 for D50/ $ 2. For the upper limit of the lower flow regime, D50/ < 2:
174



 Previous Page
Previous Page
