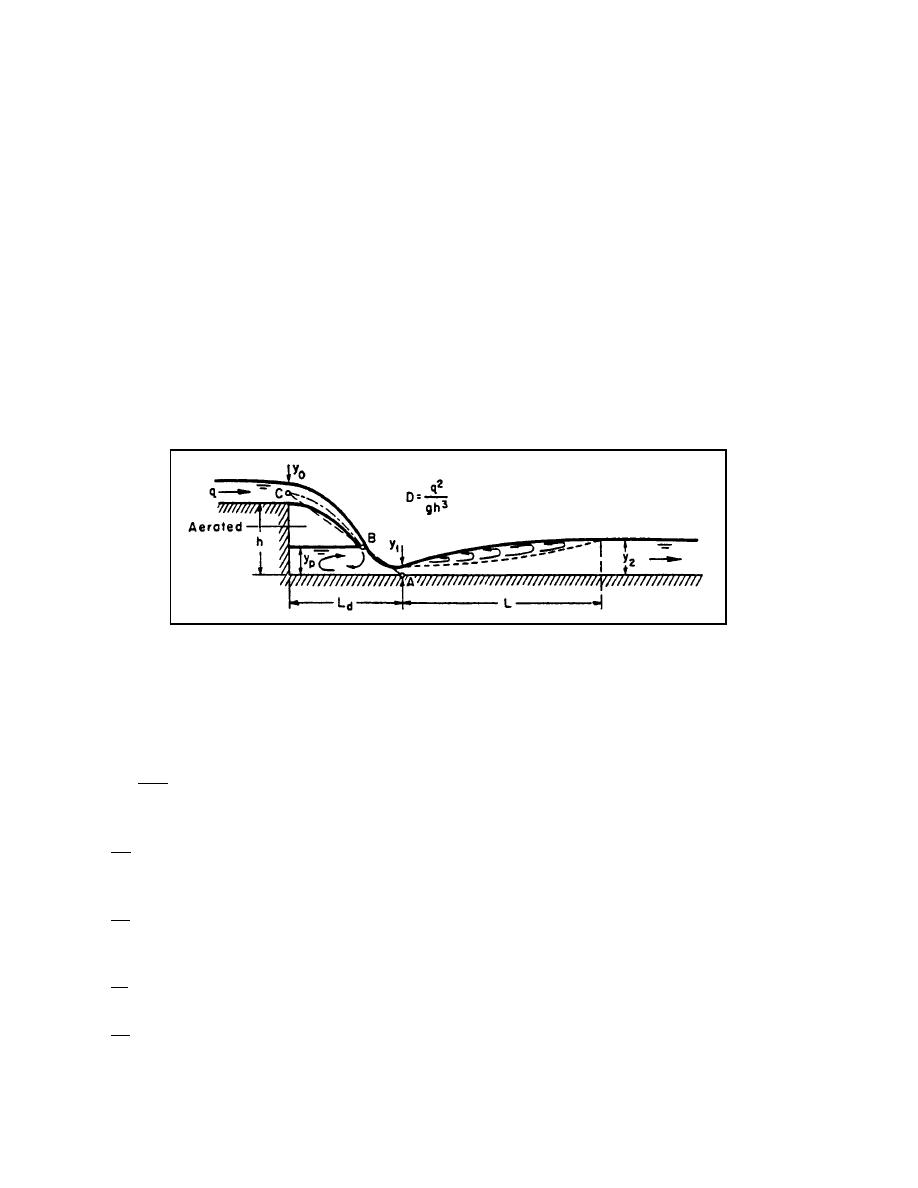
2.6.5 Flow Over Drop Structures
Subcritical flow over vertical wall drop structures at flat slopes is illustrated in Figure 2.24. As
illustrated, the flow impinging on an apron will continue downstream but at supercritical depth and
velocity. Depending on downstream conditions (bed elevation and slope and down stream flow
depth) the flow will continue to be supercritical or go through a hydraulic jump. If the flow
impinges on an erodible bed it will scour a deep hole. Methods to calculate the depth of this
scour hole are given in Chapter 3. Flow geometry and conditions of interest are:
discharge for unit width, q
drop height, h
distance from drop wall to point of impingement, Ld
depth and velocity at impingement, y1, V1
pool depth under nape, yp
sequent depth if a jump forms, y2
Figure 2.24. Flow characteristics over a drop structure (Chow 1959).
Chow (1959) gives equations to determine the geometric terms as functions of a drop number D.
In English units of measurement these are:
2
q
D=
(2.152)
g h3
LD = 4.30 D0.27
(2.153)
h
yp
= 1.00 D 0.22
(2.154)
h
y1
= 0.54 D0.425
(2.155)
h
y2
= 1.66 D0.27
(2.156)
h
2.44



 Previous Page
Previous Page
