Appendix A: A Practical Guide to Effective Discharge Calculations
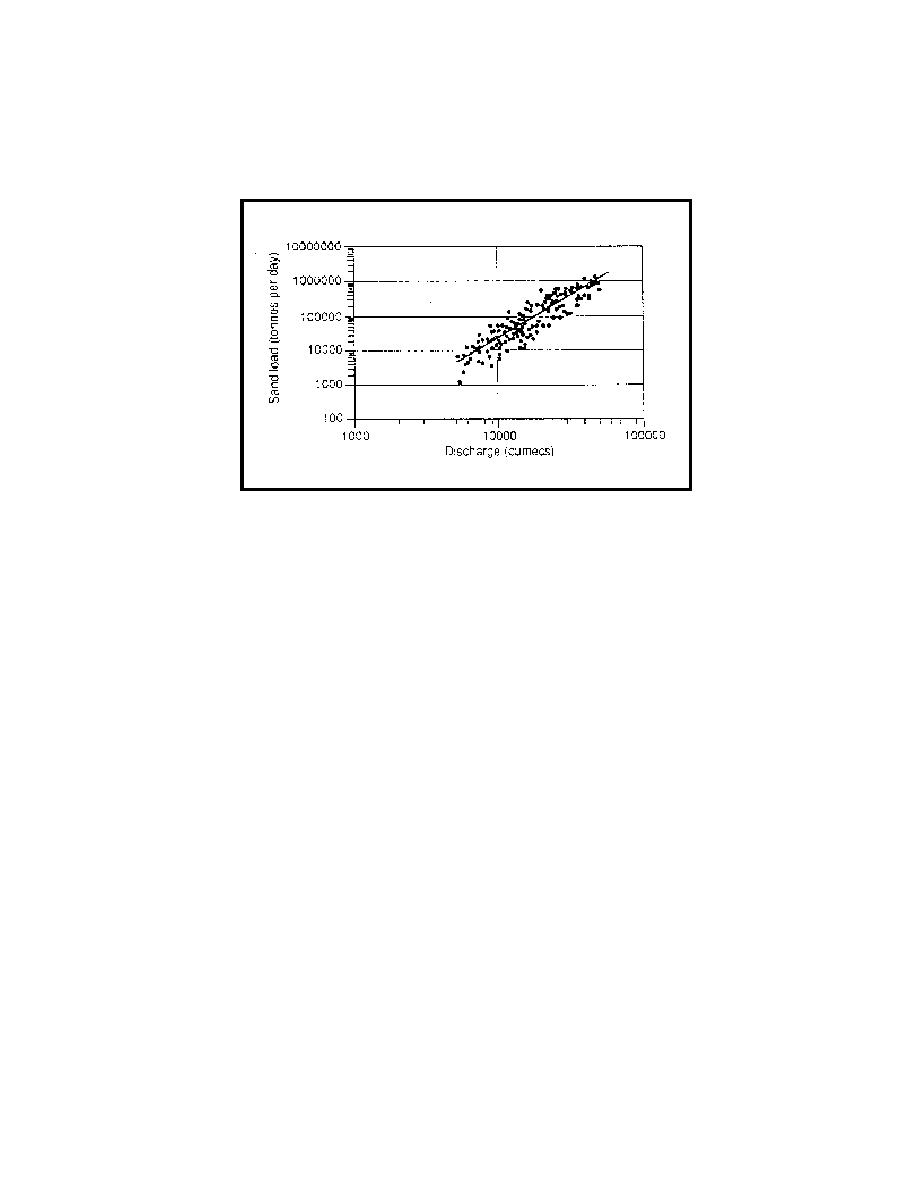
Qs = 0.00000513 Q2.42
(2)
where: Qs = sand load (tons day-1), and Q = mean daily discharge (m3s-1).
Figure 10 Sand Load Rating Curve: Lower Mississippi River at
Vicksburg for 1969-1979 (adapted from Biedenharn
and Thorne, 1994).
Bed Material Load Histogram
The data in the flow duration curve were divided into 50 equal classes ranging from 5 to 55,000
m3s-1 and with a class width of 1,000 m3s-1. The bed material transport rate for each discharge class (Qs)
was found from Eq. (2), with Q equal to the arithmetic mean discharge for that class. The quantity of bed
material load (in tons) transported by each discharge class was calculated by multiplying the frequency of
each class (in days) by the bed material transport rate for the average discharge (in tons day-1). The
resultant histogram is plotted in Figure 11.
Effective Discharge Determination
The peak of the histogram in Figure 11 is defined by the mean discharge of the modal class, which
is 30,000 m3s-1. This defines the effective discharge.
Check if Effective Discharge is Reasonable
In Biedenharn and Thorne's (1994) study, the effective discharge calculation was also performed
for gaging stations at Arkansas City (upstream of Vicksburg) and Natchez (downstream of Vicksburg).
No major tributaries enter the Mississippi between these stations. Hence, it would be expected that the
284





 Previous Page
Previous Page
