3/3/99
10/28/99
1/16/00
50
200
200
40
150
150
30
100
100
20
50
50
10
0
0
0
0
0.1
0.2
0.3
0
0.1
0.2
0.3
0
0.1
0.2
0.3
Frequency (Hz)
Frequency (Hz)
Frequency (Hz)
11/24/98
2/16/99
2/24/99
250
200
400
200
150
300
150
100
200
100
50
100
50
0
0
0
0
0.1
0.2
0.3
0
0.1
0.2
0.3
0
0.1
0.2
0.3
Frequency (Hz)
Frequency (Hz)
Frequency (Hz)
12/2/87
12/1/96
1/17/98
200
200
250
150
200
150
150
100
100
100
50
50
50
0
0
0
0
0.1
0.2
0.3
0
0.1
0.2
0.3
0
0.1
0.2
0.3
Frequency (Hz)
Frequency (Hz)
Frequency (Hz)
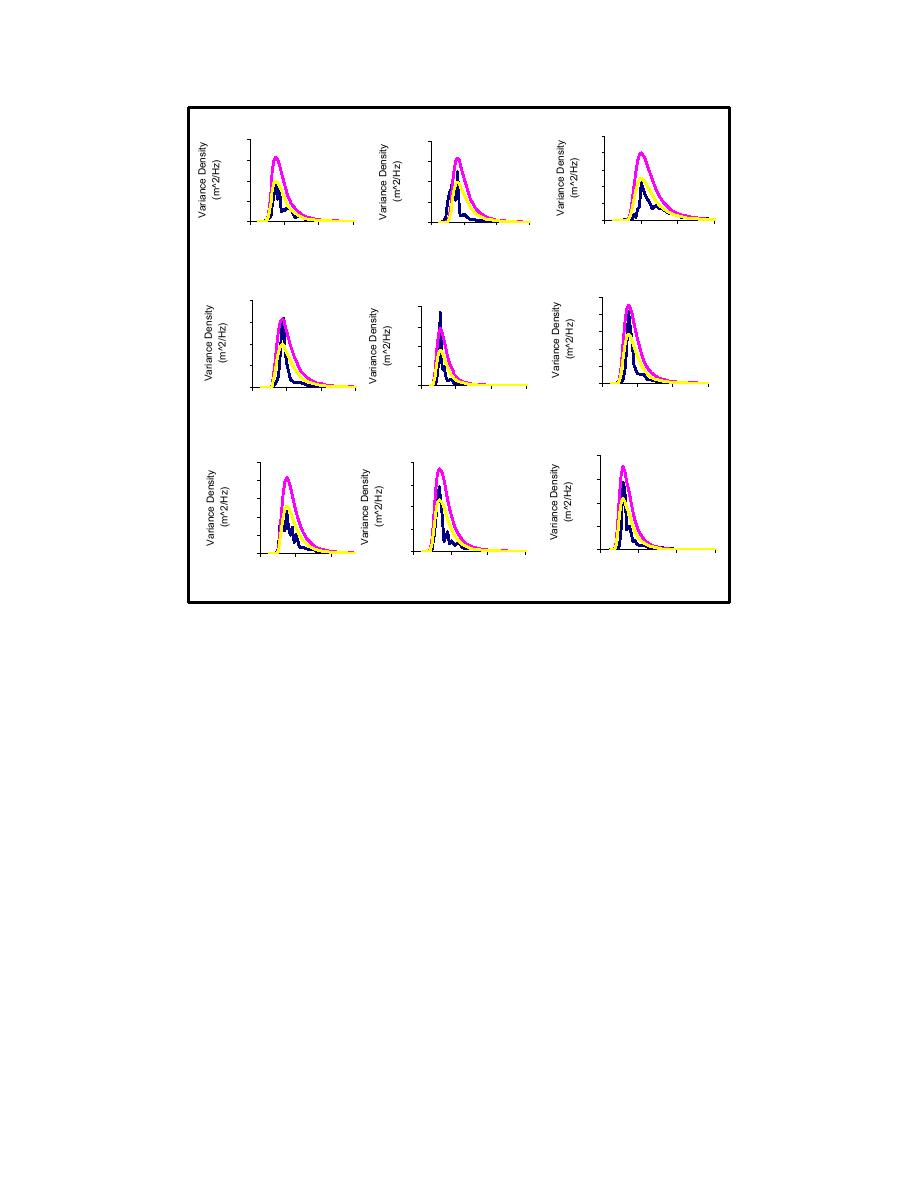
Figure A5.
Nine of 10 most extreme spectra measured at Grays Harbor CDIP
buoy between 1982-2000. Note: blue line shows measured
spectrum, yellow line is a JONSWAP spectrum with γ = 1.5 and
magenta line is a JONSWAP spectrum with γ = 3.3
Application of Existing Design Equations for
Structure Stability
Several studies have investigated rock stability for low-crested and
submerged rubble-mound structures (e.g., van der Meer 1988, van der Meer and
Pilarczyk 1990; Givler and Srenson 1986; Vidal et al. 1992; Vidal, Losada, and
Mansard 1995; Vidal, Medina, and Martin 2000; Rufin et al. 1994, 1996). Van
der Meer (1988) describes of relevant fundamental parameters for inclusion in
formulas for design of rubble-mound breakwaters and revetments: These include:
side slope of the breakwater or revetment, significant wave height, Hs deepwater
criterion, S, storm duration, often defined in terms of the number of waves, and
median mass of armor stones, M50. According to van der Meer (1988), S =
Ae/Dn50 where Ae is the area of cross-section eroded, as calculated from damaged
and undamaged profiles, and Dn50 is the nominal median armor stone diameter.
The onset of damage is generally taken as between 1 and 3. The damage
criterion, S, represents the number of stones of a nominal diameter, Dn, which are
A6
Appendix A
Stability Analysis of a Submerged Spur, North Jetty, Grays Harbor, WA





 Previous Page
Previous Page
