Assuming angle of repose φ = 40, the stability number η is obtained from Equation 6.3 or
Figure 6.14 for θ = 20 (η = 0.36). The stone size is then calculated from Equation 6.13.
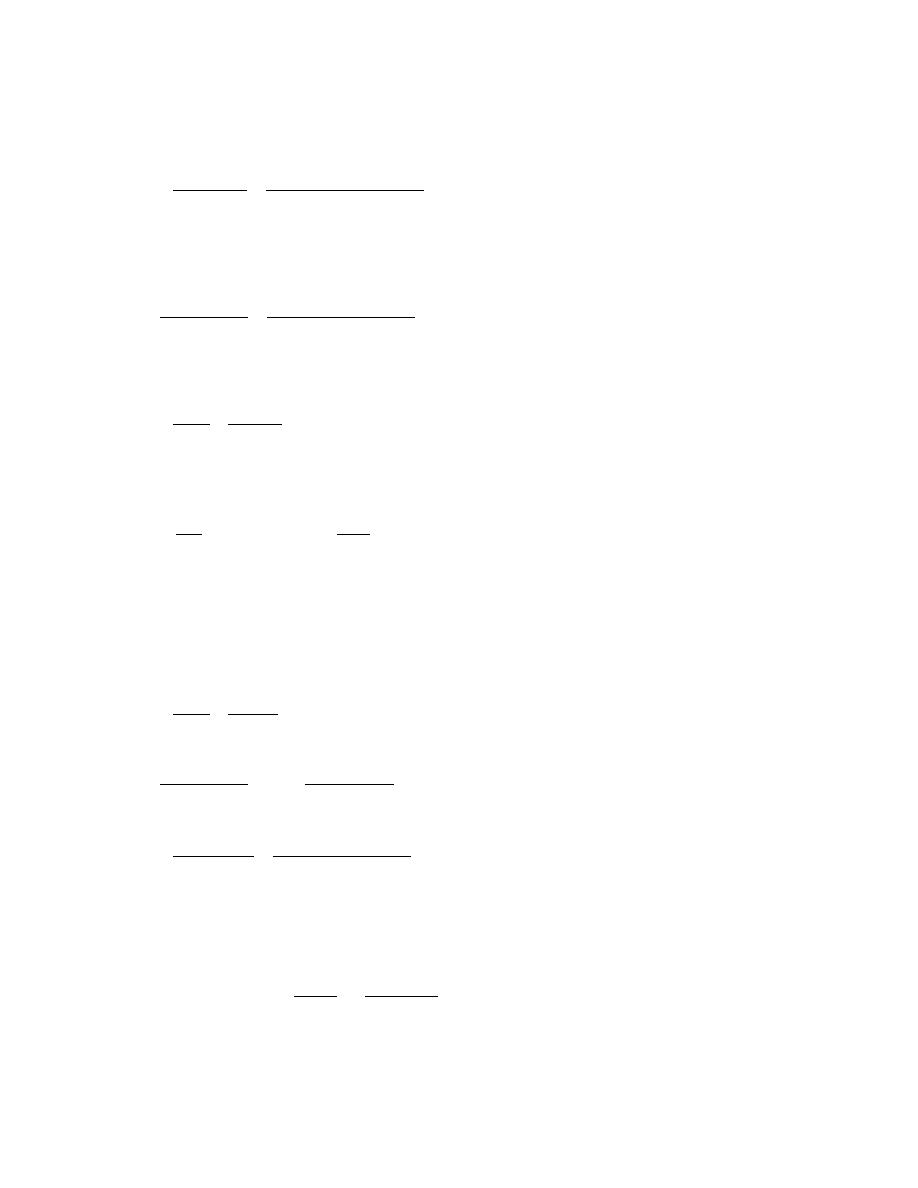
21τo
21 x 2.0
=
= 1.13 ft
Dm =
(S s - 1) γη (2.65 - 1) 62.4 x 0.36
(b) For the same design shear stress τo = 2.0 psf, determine the stability factor of particle sizes
Dm = 6 inches. From Equation 6.5
21τ o
21 x 2
η=
=
= 0.82
(S s - 1) γDm (2.65 - 1) 62.4 x 0.5
Then, Equations 6.11, 6.10, and 6.9 are used to calculate the stability factor
tan φ tan 40
Sm =
=
= 2.31
tan θ tan 20
ζ = Sm η sec θ = 2.31 x 0.82 sec 20 = 2.02
(
)
Sm
2.31
1/ 2
(ζ 2 + 4)
((2.02 2 + 4)1/ 2 - 2.02) = 0.95 < 1
S.F. =
- ζ) =
2
2
This size fraction (Dm = 6 inches) is unstable.
(c) Determine riprap size Dm for a side slope. The side slope angle θ = 20; very angular rock
with angle of repose φ = 40; Vss = 12 fps; y = 10 ft; S.F. = 1.1 and D85 / D15 = 2.0. From
Equations 6.11, 6.12, and 6.16
tan φ tan 40
Sm =
=
= 2.31
tan θ tan 20
Sm - (S.F.)2
2
2.312 = 1.12
η=
cos θ =
cos 20 = 0.66
2
1.1x 2.312
(S.F.) x Sm
0.3 V 2
0.3 x 12 2
=
=
= 1.25 ft
Dm
(S s - 1) g η 1.65 x 32.2 x 0.66
(d) Compare the size calculated in (c) with a riprap size calculated using the U.S. Army Corps
of Engineers equation (Section 6.5.4). From Equations 6.18 and 6.20:
2.5
γ 0.5
V
= S f C s C v C T y
γ - γ (K g y)0.5
D30
s
1
6.66



 Previous Page
Previous Page
