1
f ρ V2
τ=
(6.32)
8
where:
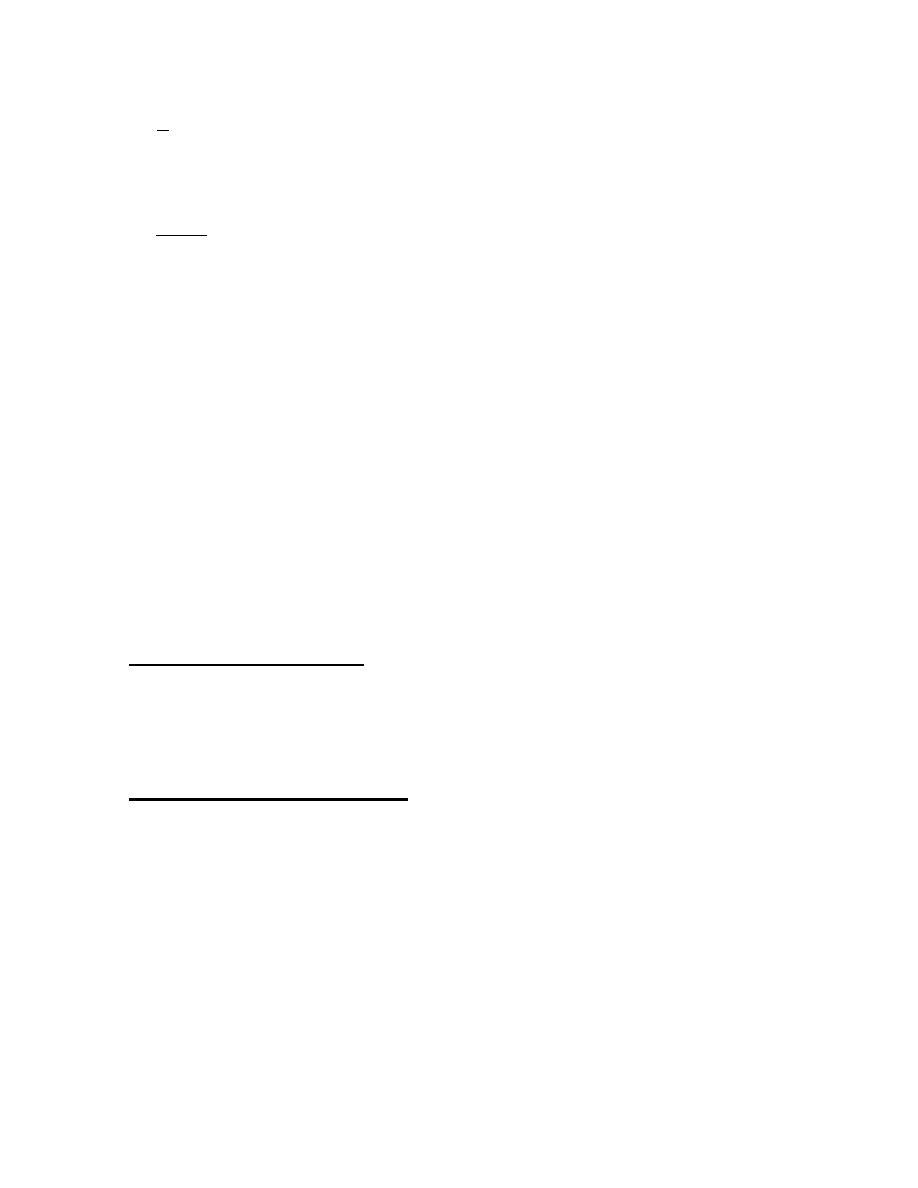
116 n2
f=
(6.33)
d1/3
and V is the local velocity, f is the Darcy-Weisbach coefficient, and ρ is the water density, n
is Manning's resistance coefficient, and d is the local flow depth. The use of the local shear
stress equation can assist in accommodating the irregular pattern of erosion and its
nonuniform progression through an embankment section at various times during an
overtopping event. This form of the shear stress equation is utilized in the program
EMBANK, described previously in this section.
6.8.4 Erosion Protection in Overtopping Flow
Materials or manufactured systems designed to protect against overtopping erosion can be
selected and designed using methods based on permissible velocity, permissible shear
stress, or both. Because velocity in and of itself is not a force, procedures based on
permissible velocity are generally derived from extensive testing and field experience using a
particular material under a variety of flow conditions, and are empirical in nature.
Procedures utilizing shear stress as a fundamental variable tend to be more theoretical in
nature, and are typically developed using a force-balance or moment-balance concept. Both
types of methods are described in this section.
Permissible Velocity Approach. Based on a battery of tests of vegetated trapezoidal
channels using various types of reinforcement materials on steep waterways, Hewlett et al
(1987) present permissible velocities for reinforced grass channels. These curves are
presented in Figure 6.30 as a function of duration of the overtopping event. Note that the
permissible velocity of concrete-based systems are independent of duration, as shown in the
figure.
Permissible Shear Stress Approach. Permissible shear stress based approaches are
usually related to force-balance (sliding) or moment-balance (overturning) representations of
the stability paradigm. Typically, a discrete-particle approach to the stability analysis is
developed using the force- or moment-balance equations for the particular system under
consideration. These approachs are presented in Hydraulic Engineering Circular No. 23
(Lagasse et al. 2001) for selected erosion control systems, e.g., articulating concrete blocks,
concrete armor units, and grout-filled fabric mats.
Laboratory or field tests are recommended in order to develop the calibration parameters
needed to fully describe the performance of the system. The permissible shear stress from
the controlled testing program is then extended to various conditions of bed slope and side
slope. Figure 6.31 shows a typical full-scale test of an articulated concrete block system in
progress under steep-slope, high-velocity flow.
6.50



 Previous Page
Previous Page
