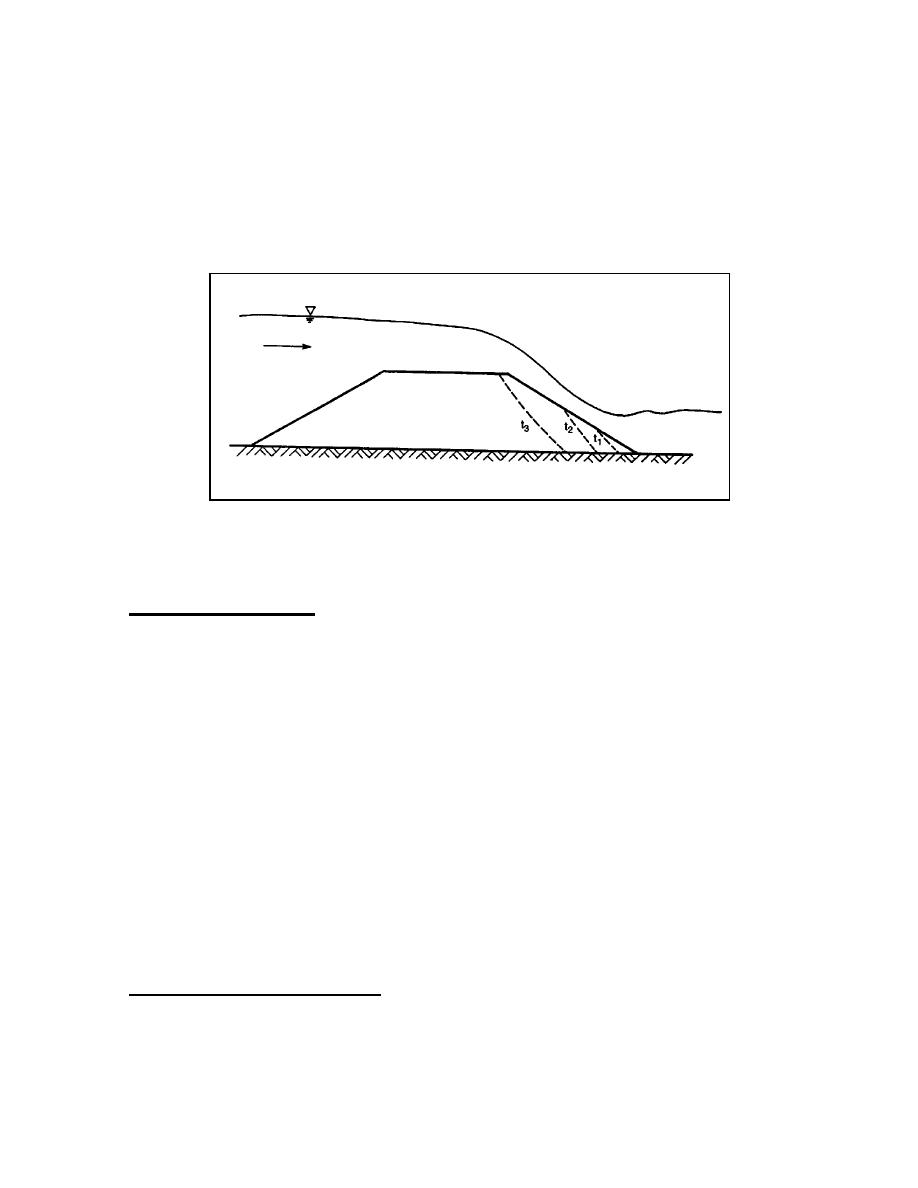
The second general erosion pattern results from the case of free flow. With low tailwater,
the flow will accelerate down the slope with high velocity and shear stress associated with
supercritical flow. Erosion typically initiates near the toe of the embankment, whether or not
a hydraulic jump is present. Erosion progresses in the upslope and upstream direction
through the embankment. Figure 6.29 (Chen and Anderson 1987) illustrates this
progression. Given sufficient duration, this type of erosion pattern typically will result in a full
breach of the embankment section.
Figure 6.29. Typical embankment erosion pattern with free flow
(Chen and Anderson 1987).
Erodibility Rate Equation. Many analytical equations have been presented to estimate the
rate of erosion under conditions of overtopping flow. Most of these equations use the
effective shear stress and/or velocity along with some measure of resistance of the
embankment material to estimate the rate of soil loss. These equations were assessed
(Chen and Anderson 1987) by relating the erosion rates measured during tests to those
calculated by the various equations.
The erodibility rate relation developed by the Agricultural Research Laboratory was found to
be the most applicable to embankment overtopping flow conditions based on these studies.
This general form of the equation is:
E = K (τ - τc )
a
(6.31)
where:
E
=
Detachment rate per unit area
τ
=
Local effective shear stress based on hydraulic conditions
τc
=
Critical shear stress of the soil
K&a
=
Empirical coefficients dependent on soil properties
Local Shear Stress Calculation.
For an embankment undergoing erosion, another
parameter of importance is local shear stress. Chen and Anderson (1987) present the
following equation (in English units) for calculating the shear stress in the immediate vicinity
of the erosion location.
6.49



 Previous Page
Previous Page
