Equation 4.48 was developed for channels with steep slopes for sand and fine gravel beds
experiencing critical and super-critical flows [Simons, et al. (1981), Julien (1995)]. The range
of parameters utilized to develop this equation is shown in Table 4.2.
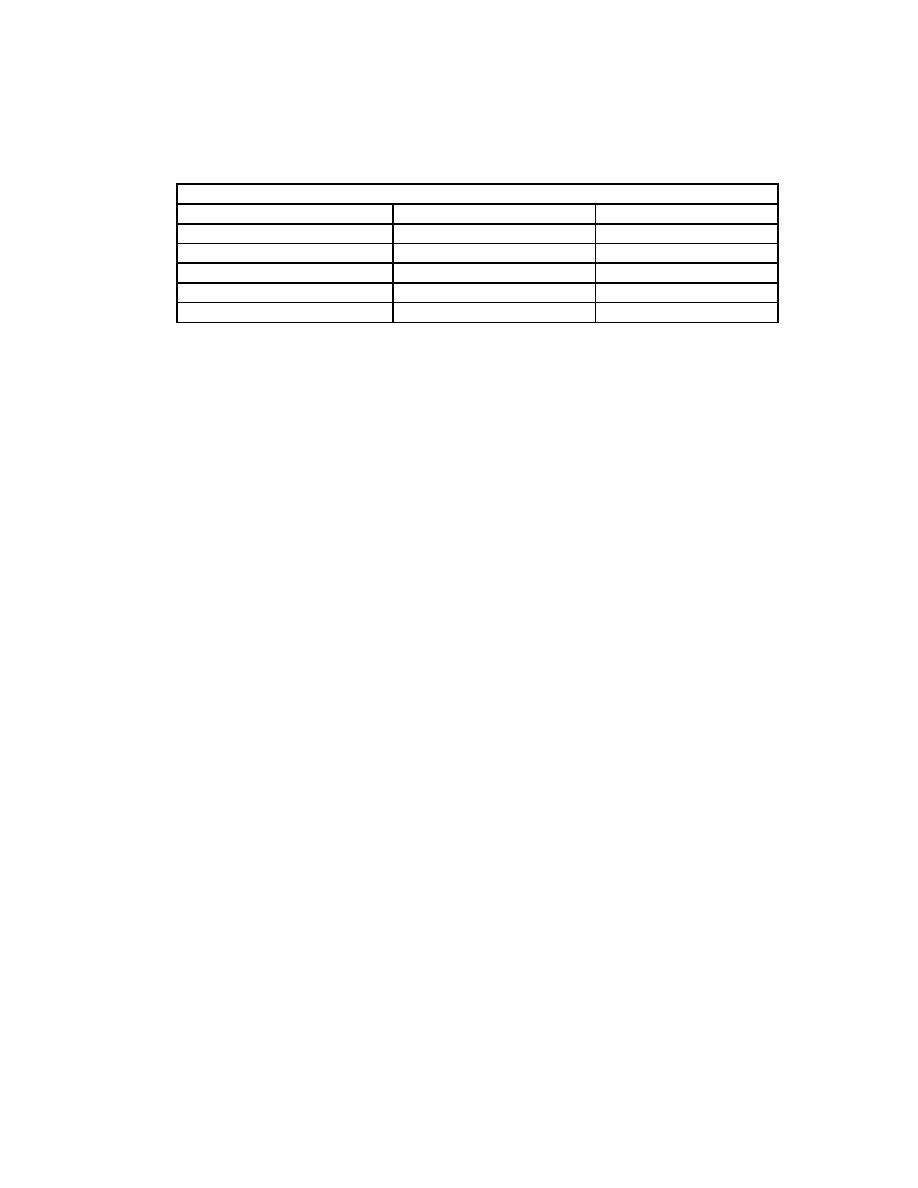
Table 4.2. Range of Parameters Equation 4.48 Developed by Simons et al.
Parameter
Value Range
SI Units
Froude Number
14
--
Velocity
1.98 7.92
m/s
Bed Slope
0.005 0.040
m/m
m2/s
Unit Discharge, q
3.05 60.96
Particle Size, D50
> 0.062
mm
As the depth increases for a given velocity, the intensity of the turbulent transfer properties
decreases for these sizes. The increase in area available for suspended sediment with the
increased depth does not totally counterbalance the reduced turbulent transfer
characteristics. The result is an inverse dependence of transport rate on depth for these
larger sizes. The sizes with no dependence (Cs2 = 0) on depth in their transport rate fall
between these two extremes.
When applying the equations resulting from Table 4.1, care should be taken to assure that
the range of parameters being used is not out of range with those used to develop the
equations (Table 4.2). If conditions are within the ranges outlined in Table 4.2 the regression
equations should provide results within ten percent of the values computed using the Meyer-
Peter and Mller bedload and Einstein suspended load equations for the steep conditions in
Table 4.2.
There are several other checks that should be made in order to ensure the equations are
applicable to a given problem. The equations are based on the assumption that all the
sediment sizes present can be moved by the flow. If this is not true, armoring will take place.
The equations are not applicable when armoring occurs. Since the equations were
developed for sand-bed channels, they do not apply to conditions when the bed material is
cohesive. Equation 4.48 would overpredict transport rates in a cohesive channel.
An example of the application of the basic power function relationship is given in Section
4.12 (SI) and Section 4.13 (English).
4.6.3 Expanded Power Function Relationship
Kodoatie et al. (1999) modified a Posada (1995) equation using nonlinear optimization and
the field data for different sizes of riverbed sediment. A description of the development and
validation of this equation is presented in Appendix B. The resulting equation is:
qt = aV b y c S d
(4.50)
where:
qt
=
Sediment transport rate, metric tons/m/day (tons/ft/day)
V
=
Mean flow velocity, m/s (ft/s)
y
=
Mean flow depth, m (ft)
S
=
Energy slope
a, b, c, and d
=
Regression coefficients
4.29



 Previous Page
Previous Page
