D-R-A-F-T
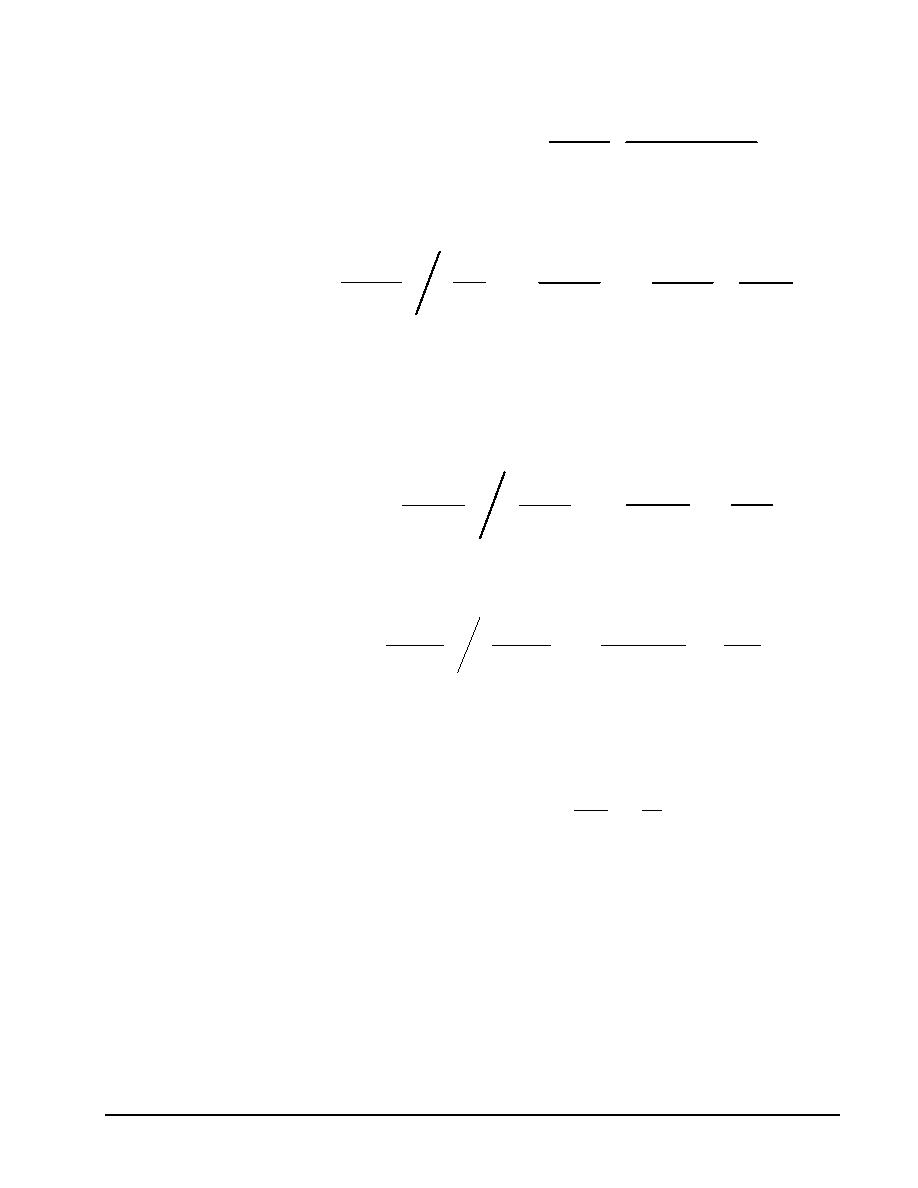
⎛ C oV ⎞ ( C '- G p ' )V m '
⎜L H ⎟
α 1C + α 2 = - ⎜
⎟
D'
⎠
⎝ er
(sand erosion/storage). The sand deposition to storage ratio is
Equation 43
⎞⎛ V s ∆ t ⎞
⎞ ⎛ V
⎛ C o ⎞ ⎛ V∆t
⎛ C oV ⎞
⎟⎜
⎟=⎜
⎟=⎜
⎜
⎜L H ⎟
⎟ H ⎟ = VrC v
⎜
⎟
⎟ ⎜V L
⎝ ∆ t ⎠ ⎜ Ler H
⎠
⎠⎝
⎝
⎠
⎠ ⎝ s er
⎝
er
The term Lde ,already dimensionless, so that this non-dimensional term is the vertical
advective Courant number multiplied by a velocity ratio, Vr, of the current velocity
and the fall velocity times the entrainment length factor Ler.
(sand erosion/convection). The ratio of sand deposition to convection is
Equation 44
⎞ C ve
⎛ C oV ⎞ ⎛ L
⎛ C oV ⎞
⎟=
⎟=⎜
⎜L H ⎟
⎜
⎜
⎟
⎟ C
⎝ L ⎠ ⎜ Ler H
⎝ er ⎠
⎠
⎝
n
(sand erosion/diffusion). The ratio of sand deposition to diffusion is
Equation 45
⎛ C o D r ⎞ ⎛ VL
⎞ C ve
⎛ C oV ⎞
2
⎟=⎜
⎟=
⎜L H ⎟
⎜
⎜
Pe
⎟
⎠ ⎜ D r L er H
⎟
2
⎝ L
Cn
⎝ er
⎠
⎝
⎠
Clay Deposition
Clay deposition in SED2D is represented by
Equation 46
VseC ⎛
⎞
τ
α1C + α 2 =
⎜1 -
⎟
D ⎜ τd
⎟
⎝
⎠
where:
bottom shear stress, N-m/sec2
τ
=
critical bottom shear stress for deposition, N-m/sec2
τd
=
Vse
=
the effective fall velocity, m/sec
These terms will all scale as before and the term in parentheses that includes the ratio
of shear stresses is already non-dimensional. The effective fall velocity is used for
clay deposition because for clay the relationship between concentration and fall
velocity, if the option is used in the program, is nonlinear.
Equation 47
48 Advanced Techniques
Users Guide To SED2D-WES



 Previous Page
Previous Page
