D-R-A-F-T
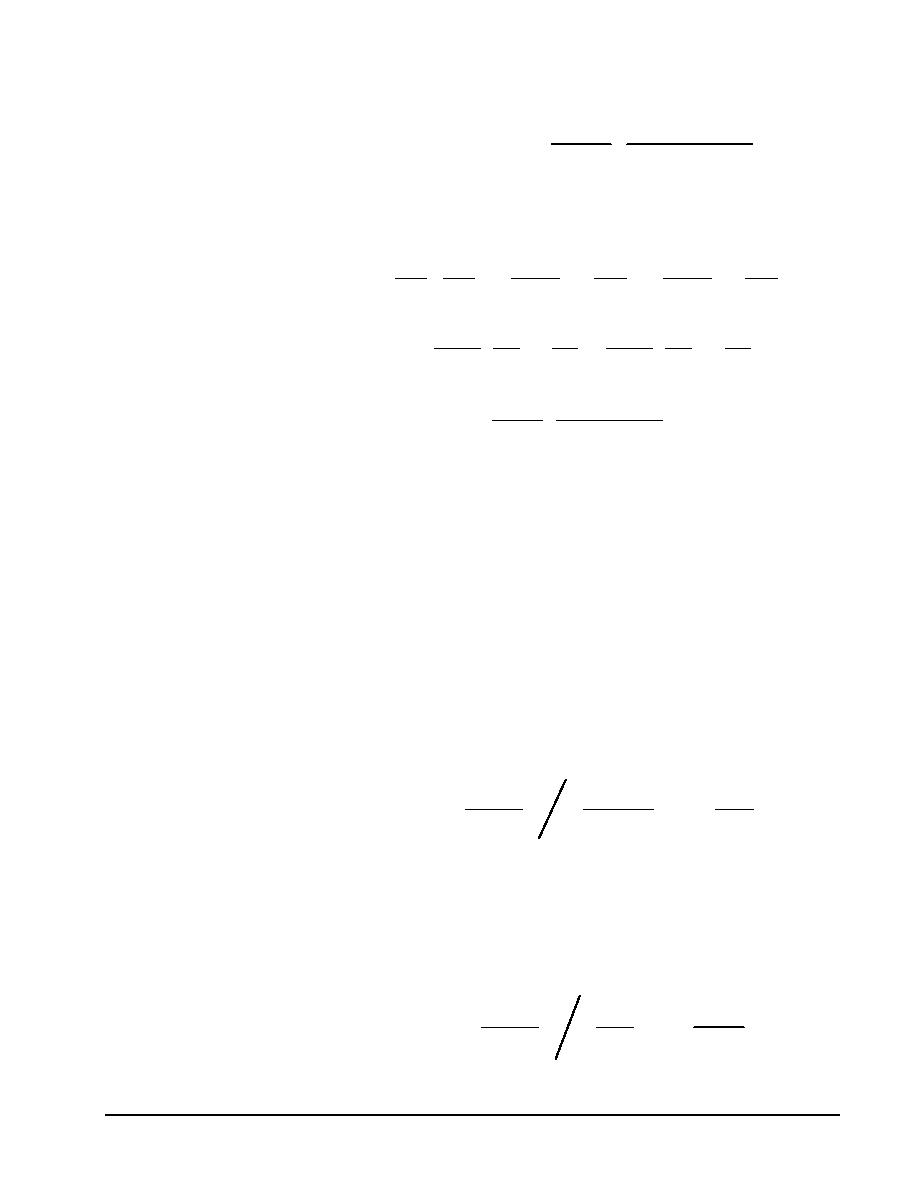
⎛ C oVs ⎞ ( C '- G p ' )V s '
α 1C + α 2 = - ⎜
⎜L H⎟
⎟
D'
⎝ de ⎠
The full equation for sand deposition becomes
Equation 32
⎛ C 0 ⎞ ∂ c ' ⎛ C 0V ⎞ ∂ c ' ⎛ C 0V ⎞ ∂ c '
+⎜
+⎜
⎜
⎟
⎟u '
⎟v'
⎝ ∆ t ⎠ ∂t ' ⎝ L ⎠ ∂x ' ⎝ L ⎠ ∂y '
⎛DC ⎞ ∂
∂c' ⎛ DrC0 ⎞ ∂
∂c'
-⎜ r2 0 ⎟
-⎜ 2 ⎟
Dx '
Dy '
⎝ L ⎠ ∂x'
∂x' ⎝ L ⎠ ∂y'
∂y'
⎛ CoVs ⎞ (C '-G p ' )Vs '
-⎜
⎜L H⎟
=0
⎟
D'
⎝ de ⎠
where the first term is storage, the second and third terms are x- and y- advection, the
fourth and fifth terms are x- and y-diffusion, and the last term is the bed sink (sand
deposition)
These terms in the equation above represent the various aspects of the transport
process. Note that the terms with the primed variables have been scaled such that
these terms are non-dimensional, taking on the scale of unity. The bolded terms now
contain the dimensional characteristics of the process. The normal method for the
interpretation of these scaling factors is to develop the ratios of these terms.
There are several dimensional groupings that are independent of the bed source-sink
variables. The ratios of these terms will be addressed first.
Advection/Diffusion Ratio
For example, the ratio of the advective terms to the diffusion terms yields the Peclet
Number
Equation 33
⎛ VL
⎞
⎛ C 0Dr ⎞
⎛C V ⎞
⎟= ⎜
⎟
Pe = ⎜ 0 ⎟
⎜
⎜ D
⎟
2
⎝ L ⎠
⎝ L
⎠
⎝
⎠
r
This parameter has been used extensively to deal with numerical oscillations caused
by over-advecting the solution.
Advection/Storage Ratio
Another basic parameter is the ratio of the advection and storage term
Equation 34
⎛ C o ⎞ ⎛ V∆t ⎞
⎛ C oV ⎞
Cn = ⎜
⎟=⎜
⎟
⎟
⎜
⎝ ∆t ⎠ ⎝ L ⎠
⎝ L ⎠
Advanced Techniques 45
Users Guide To SED2D-WES



 Previous Page
Previous Page
