=
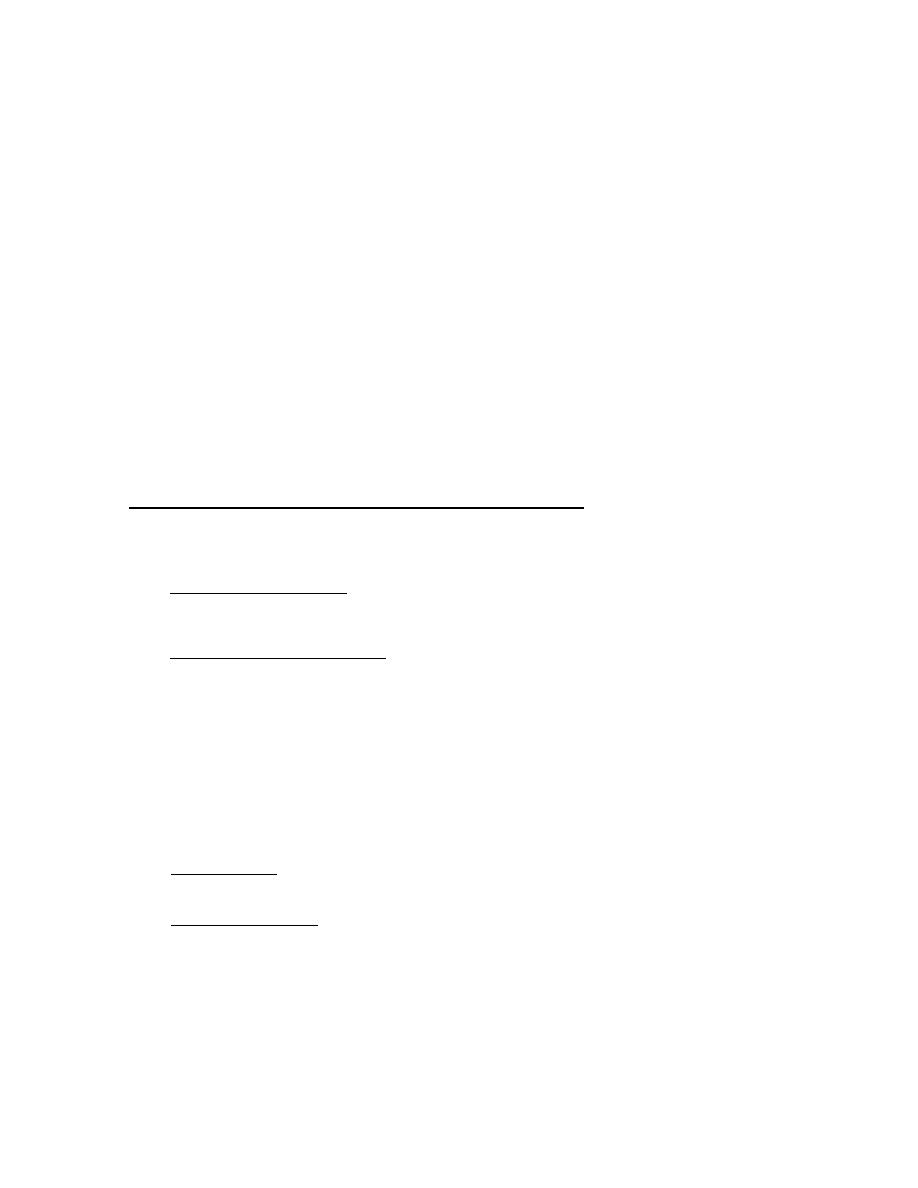
Specific gravity (2.65 for quartz)
Ss
Density of water (999 kg/m3, 1.94 slugs/ ft3)
=
ρ
Density of sediment (quartz 2,647 Kg/m3, 165.4 lb/ft3)
=
ρs
g
=
The relationships in Equations 3.43 and 3.44 are the fundamental relations between velocity,
depth, resistance to flow (Manning's n), density and a coefficient determined experimentally
for the beginning of movement of the sediment particles. This coefficient is called the
Shields coefficient. The equation can be solved for the following:
1. Critical velocity for beginning of sediment movement for a given depth, roughness,
Shields coefficient, and bed material size and density.
2. Critical size for a given velocity, depth, roughness, Shields coefficient, and bed material
density.
3. Clear-water scour depth for a given velocity, roughness, Shields coefficient, and bed
material size and density. This depth is the contraction scour depth at the end of a long
contraction.
Critical Velocity for the Beginning of Bed Material Movement. Rearranging Equations 3.43
and 3.44 to give the critical velocity for beginning of motion of bed material of size D for
depth y, Shield's parameter, and Manning's n results in:
K 1/ 2 (S s - 1)
1/ 2
D1/ 2 y1/ 6
Vc = s
s
(SI)
(3.45)
n
1.49 k 1/ 2 (S s - 1)1/ 2 D1/ 2 y1/ 6
s
s
Vc =
(English)
(3.46)
n
n = K nu D1/ 6
(3.47)
s
Knu = 0.041
(SI)
Knu = 0.0336
(English)
Vc = K u D1/ 3 y1/ 6
(3.48)
s
K 1/ 2 (S s - 1)
1/ 2
Ku = s
(SI)
(3.49)
K nu
1.49 K 1/ 2 (S s - 1)1/ 2
s
Ku =
(English)
K nu
where:
=
Critical velocity above which bed material of size D and smaller will be
Vc
transported, m/s, ft/s
=
Shields parameter
Ks
3.41



 Previous Page
Previous Page
