Hivel2D users manual
σ xx ,σ xy ,σ yx ,σ yy = Reynolds stresses where the first subscript indicates the direction and
the second indicates the axis direction normal to the face on which the
stress acts
ρ = fluid density
z0 = channel invert elevation
n = Manning's roughness coefficient
C0 = dimensional constant (C0 = 1 for SI units and C0 = 1.486
for English units)
The Reynolds stresses are determined using the Boussinesq approach relating stress to the
gradient in the mean currents:
u
σ xx = 2 ρ ν t
x
u
v
= ρνt
σ xy = σ yx
+
(6)
x
y
v
= 2ρ νt
σ
yy
y
where nt is the eddy viscosity, which varies spatially and is solved empirically as a function
of local flow variables (Rodi 1980):
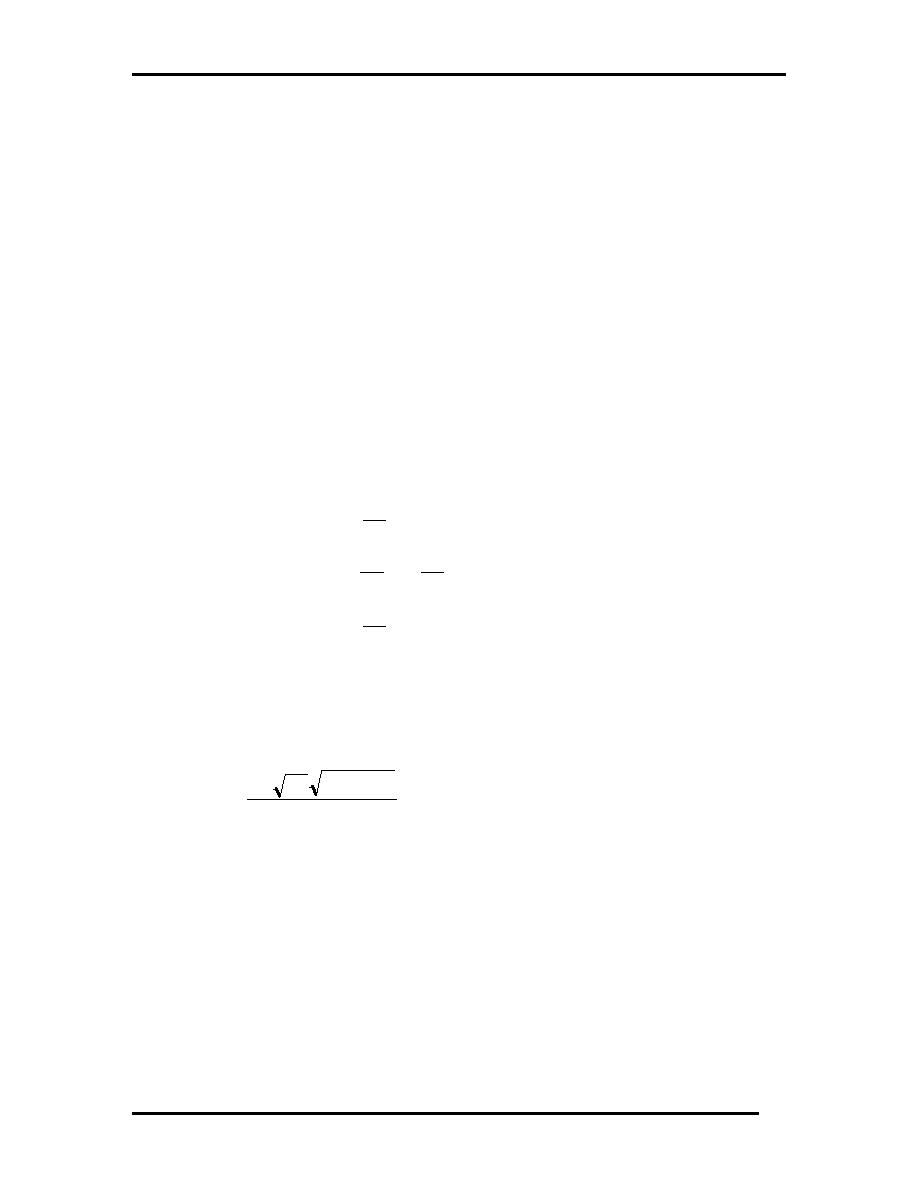
2
2
Cn 8g p + q
νt
=
(7)
C 0 h1/ 6
where C is a coefficient that varies between 0.1 and 1.0.
The typical high-velocity channel is rather wide and shallow, so that the bulk of the
turbulence is bed-generated and therefore Equation 7 is reasonable. However, if the walls
or other structures make a significant contribution to the overall turbulence, this equation
will underpredict the magnitude of the eddy viscosity or turbulence. Even under typical
channel configurations there is fairly large uncertainty in the parameter C. This is
apparent since it has been found to range from 0.1 to 1.0. Therefore, the user should
consider making a sensitivity check on the calculated water surface for a range of values
of C and any other parameters having values that are largely uncertain.
4
Chapter 2 HIVEL 2D overview





 Previous Page
Previous Page
