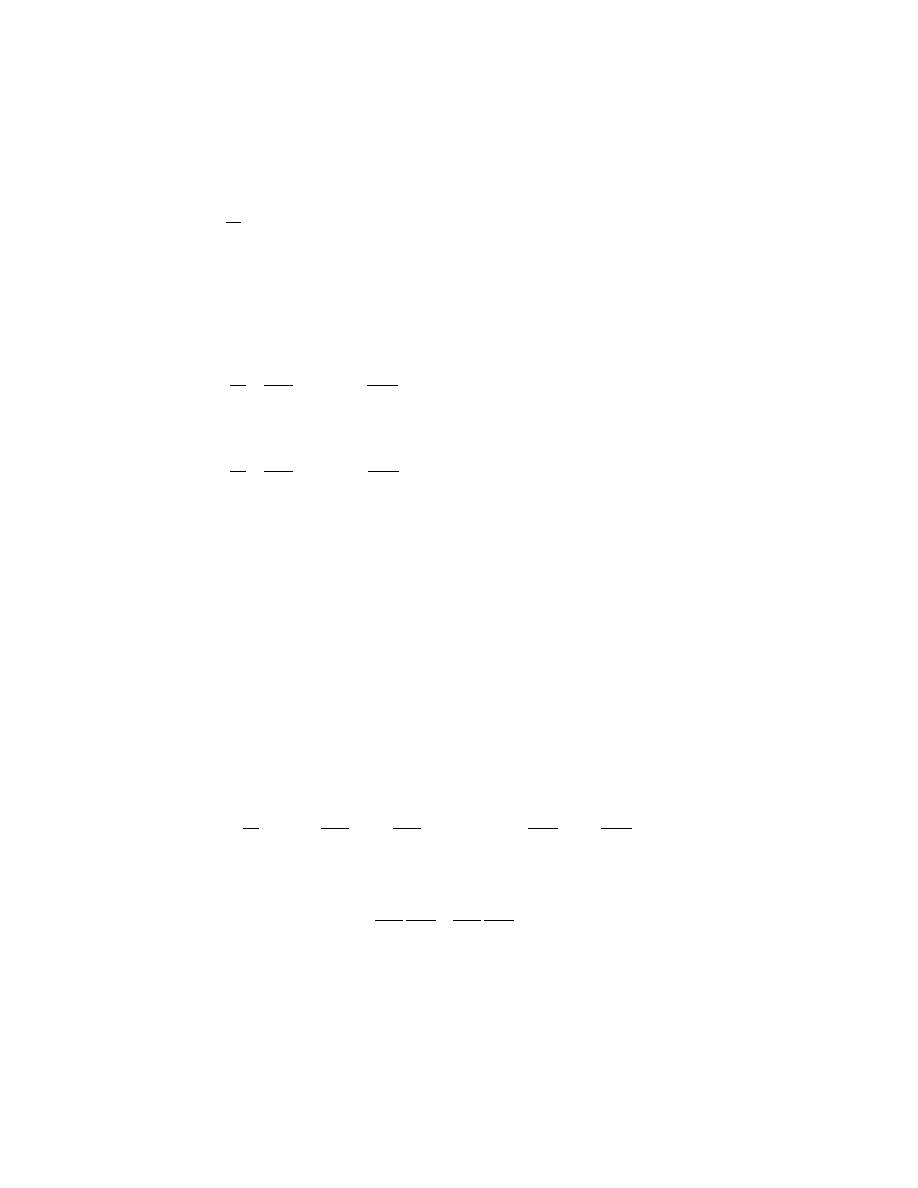
$
$
parts on η , and replacing -π/2-ωt with α; this gives
$
g
[ 1 cos α + η2 sin α ]
Φ=
η
(127)
Z
ω
An expression for the velocity of water particles is obtained by evaluating the
gradient of the expression for Φ in the last equation; this gives
η1
η
^
^
g
vx =
cosα + 2 sin α Z
(128a)
x
ω
x
η1
η
^
^
g
cos α + 2 sin α Z
vy =
(128b)
y
ω
y
These expressions contain the horizontal components of the velocity. For simplicity, Z is
taken as a local constant.
The magnitude of the horizontal components of the velocity is obtained by
substituting vx and vy from the last expression into
|v|2 = (vx)2 + (vy)2
(129)
to obtain
g 2 η1 η1 2
η 2 ηy 2 2
2
2
$ 2
$
$
$2
| v|2 = Z +
cos α +
sin α
+
ω
x y
x y
η1 η2
η η
$$
$$
sin (2α )
- 1 2+
(130)
y y
x x
The maximum horizontal velocity occurs at locations where the derivative of |v|2
with respect to α is equal to zero; this occurs where
38



 Previous Page
Previous Page
