C
(
)
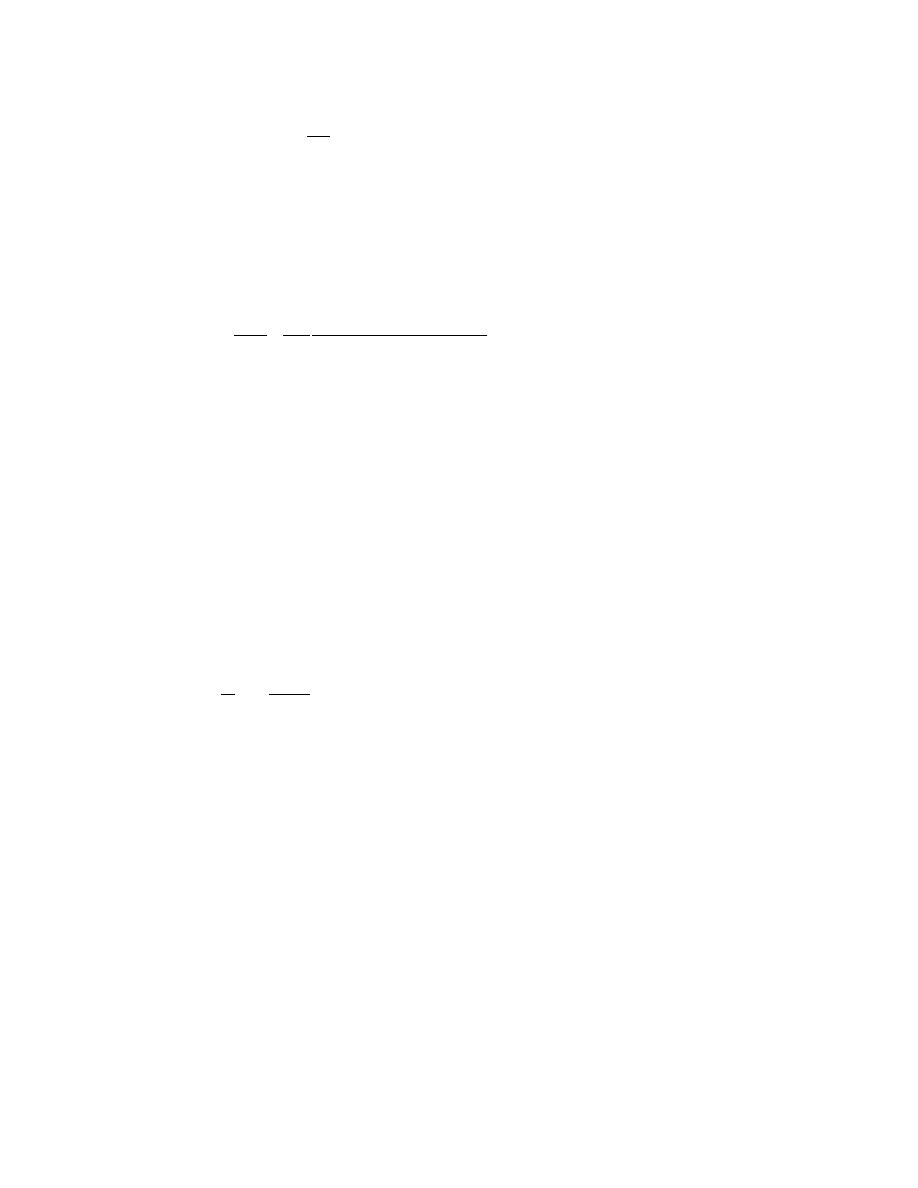
$ + g σ2 + iσw + iCg σγη = 0
∇ ⋅ CCg ∇ η
(4)
$
C
where w is a friction factor and γs a wave breaking parameter. Following Dalrymple et al.
i
(1984), we have used the following form of the damping factor in CGWAVE:
2nσ2f r
ak 2
w=
(5)
k 3π (2kd + sinh 2kd )sinh kd
user. The coefficient fr depends on the Reynolds number and the bottom roughness and
may be obtained from Madsen (1976) and Dalrymple et al. (1984). Typically, values for fr
s
n.
function of (x,y) allows the modeler to assign larger values for elements near harbor
entrances to simulate entrance loss. For the wave breaking parameter γ we use the
,
following formulation (Dally et al 1985, Demirbilek 1994, Demirbilek et al. 1996b):
χ Γ2 d 2
γ 1 -
=
(6)
d
4a 2
where χ is a constant (a value of 0.15 is used in CGWAVE following Dally et al (1985))
and Γ is an empirical constant (a value of 0.4 is used in CGWAVE).
In addition to the above mechanisms, nonlinear waves may be simulated in the
MSE. This is accomplished by incorporating amplitude-dependent wave dispersion, which
has been shown to be important in certain situations (Kirby and Dalrymple 1986). The
nonlinear dispersion relation used in place of Equation 3 is
7



 Previous Page
Previous Page
